How to Solve Rebus Puzzles With the Help of 7 Examples With Solutions

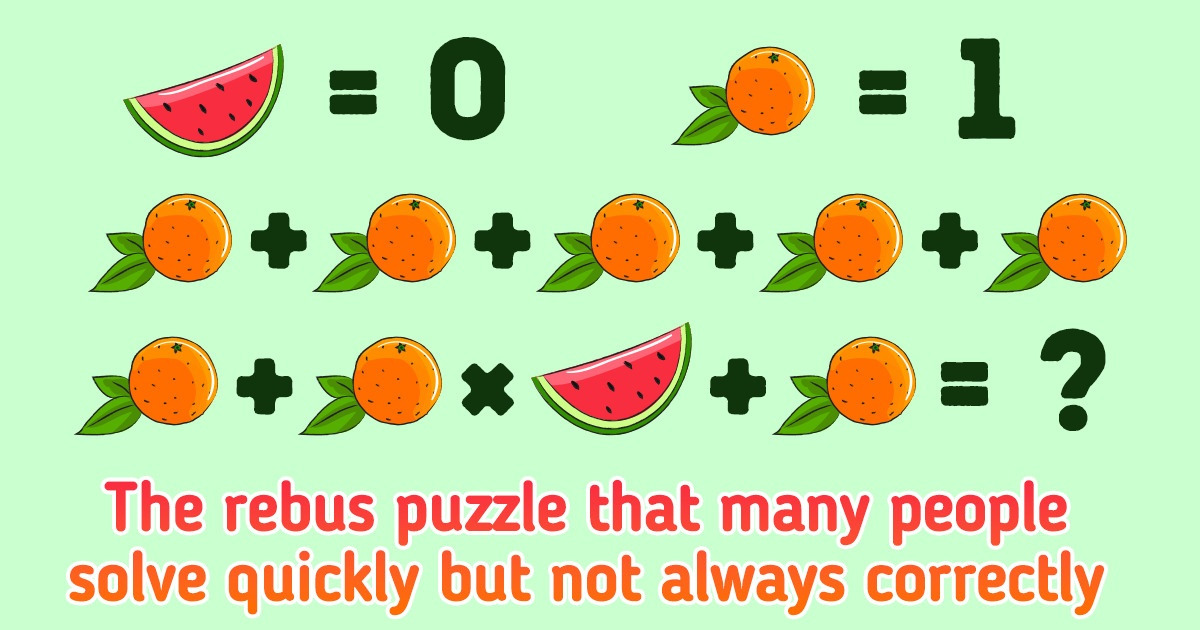
Mathematical rebuses are graphical puzzles in which numbers and arithmetic operations are encrypted. To solve them, one normally needs to use logical thinking and savvy. Moreover, they develop non-standard thinking because a mysterious picture can be interpreted differently while searching for the answer.

5-Minute Crafts is telling you how to learn to solve rebus puzzles of different degrees of difficulty quickly.

In what ways mathematical rebus puzzles are different from normal rebus puzzles

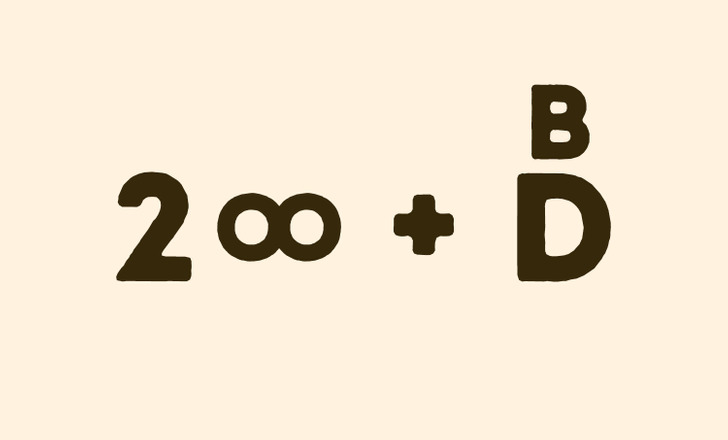
A rebus puzzle is a brain teaser where a word or a phrase is encrypted with the help of pictures and words. Some linguists believe that this kind of riddle formed the basis for the appearance of hieroglyphs in several languages. In the eighteenth and nineteenth centuries, people often used puzzles to encrypt individual words in correspondence.

A mathematical rebus is also a brain teaser with pictures and numbers. But in order to solve it, one needs to use a mathematical approach or to recall the algebraic language. For example, look at the picture above. It has an English phrase encrypted in it. If you say the names of the symbols from the left to right, you’ll get: 2 (the number two) + Infinity (the symbol of infinity) + Beyond ( B on D) — to Infinity and Beyond.

This brings us to the conclusion that any picture or symbol in a rebus has some role in solving the puzzle.

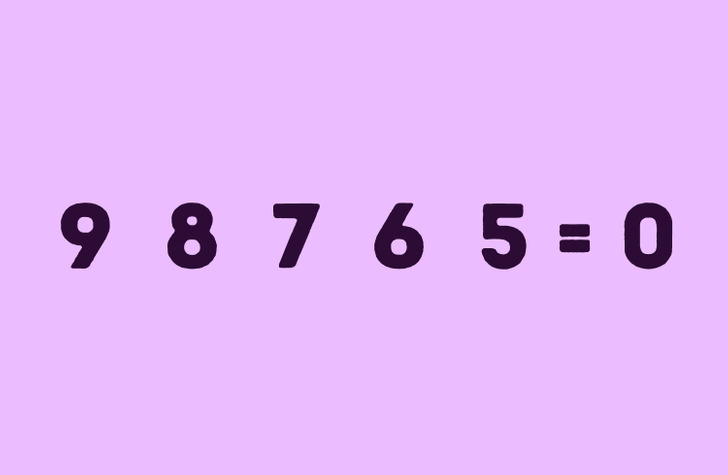
So in order to solve some rebuses, it’s important to know the language they are made in. To solve the rebus above, you need to know the 4 main arithmetic operations (addition, subtraction, multiplication, and division) and apply logical thinking.

Therefore, in the left part of the equation, you need to place mathematical signs between the numbers so that the result is zero on the right side. The solution: 9 × 8 − 7 − 65 = 0.

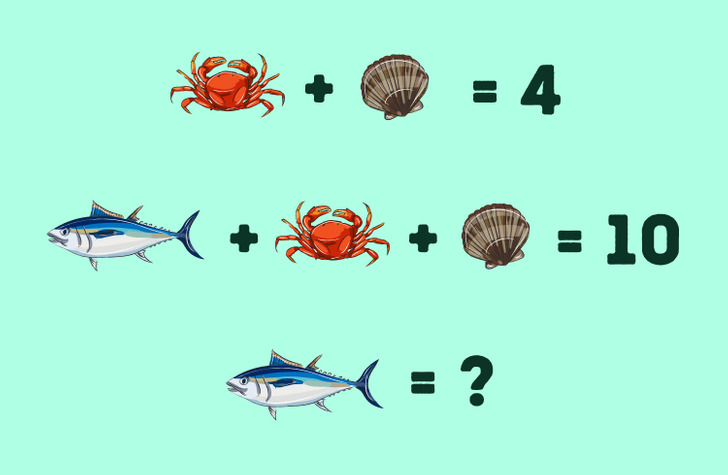
A rebus can hide a numerical expression. In the picture above, there is a simple puzzle encrypted — it has 2 equations with unknowns. In order to solve it, we need to take into account that all the equations are connected with each other, while the pictures are hiding integers not equal to zero.

In the first equation, 1 crab and 1 seashell make 4. It means we are talking about 2 different addendums that make up 4. It’s quite easy to find them — 3 and 1. In order to know what the fish equals, we need to subtract the sum of the known 2 terms from the sum of 3 terms. We get: 10 − 4 = 6. It means the fish equals 6.

After having figured out how to solve such a simple rebus, we can move to one that is more complicated.

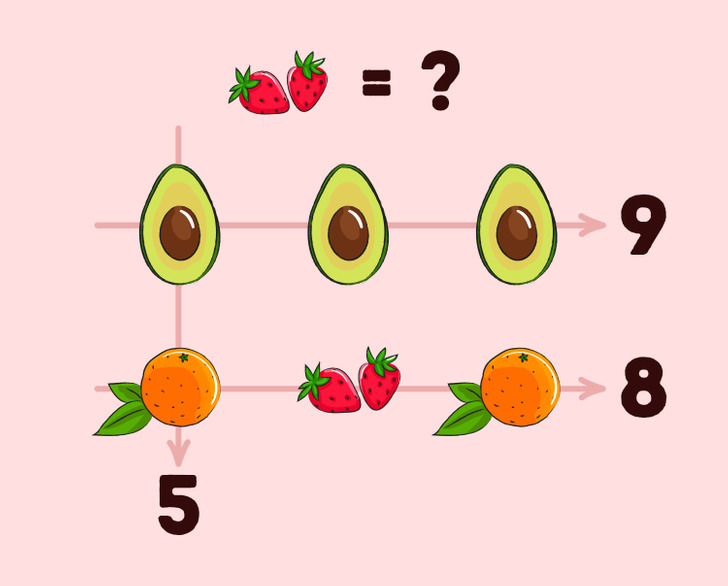
Such puzzles can look different. For example, the picture above shows a chart with avocados, strawberries, and oranges.

• If you sum up all avocados in the first row, you’ll get 9.
• If you sum up all berries and fruits in the second row, you’ll get 8.
• If you sum up all avocados and oranges in the first column, you’ll get 5.

You need to find out what strawberries are equal to. This puzzle looks like the previous one but it is illustrated a bit differently and is a bit more complicated. If you solve it correctly, you’ll get 4: avocado equals 3, while an orange equals 2.

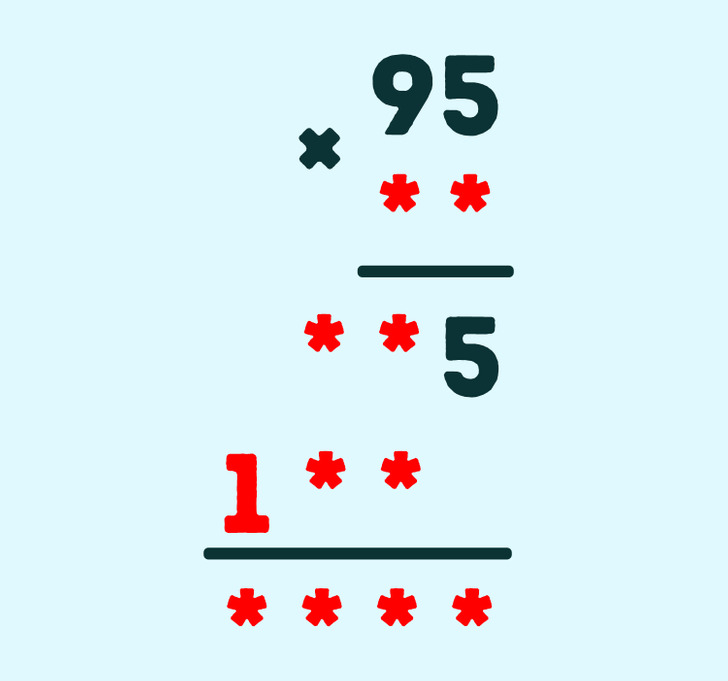
A math rebus can also look like a regular numerical equation where, instead of numbers, there are stars or dots as is shown in the picture above. In the case of this example with multiplication by a column, the method of selecting products and sums, as well as logical thinking, will help. The 2 first stars are the number 23, then it’s 285 and 190, and the last number is 2 185. Eventually, we get, 95 × 23 = 2 185.

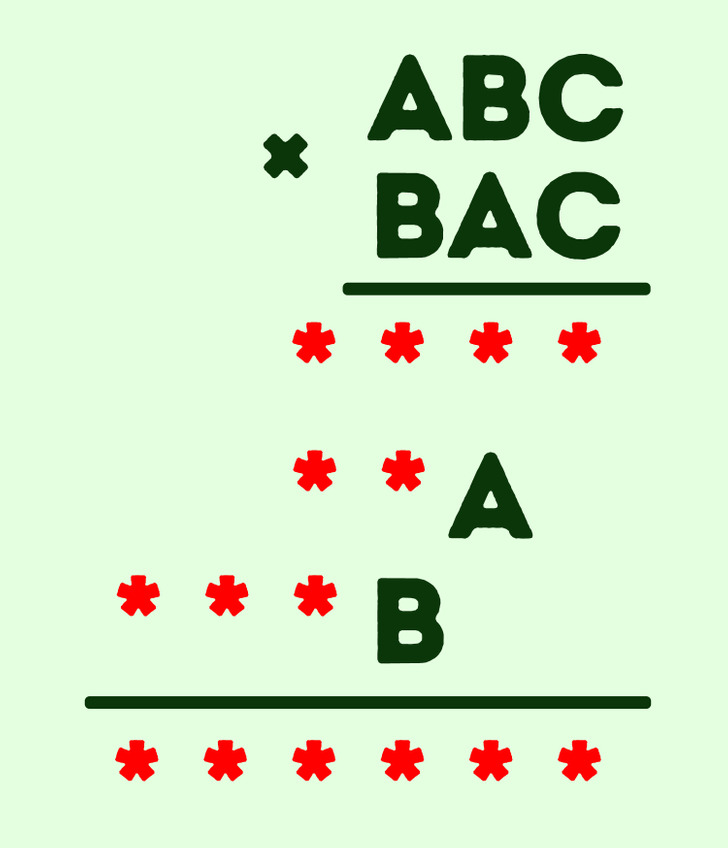
This rebus can be complicated by replacing numbers with letters. In the picture above, multiplication of two 3-digit numbers by a column is encrypted. Let’s look closer at the solution:

• Look at line 4 where we have the result of ABC × A multiplication. If the last digit of this product equals A, it means it is not 1, otherwise, C would also equal 1. At the same time, A can’t be bigger than 3, otherwise, the product would have a 4-digit number. It means A = 2 or A = 3.
• If A = 3, C should equal 1. But it’s not correct because ABC × С makes up a 4-digit number in line 3. It means A = 2, while A × C should give the number that ends with 2. It means C = 6.
• Let’s look at line 5 where we have the result of ABC × B or 2B6 × B. The last digit of this product equals B and we also get it with 6 × B. It means B can be hiding either 4 or 8. In the first case, we will have 246 × 4 = 984, which doesn’t correspond to a 4-digit product. It means B = 8.

All in all, A = 2, B = 8, and C = 6. Therefore, 286 × 826 = 236 236. In line 3 we get 1 716, in line 4 we get 572, in line 5 we get 2 288. The rebus is done!

Bonus: a simple rebus puzzle that many people solve quickly
but not always correctly

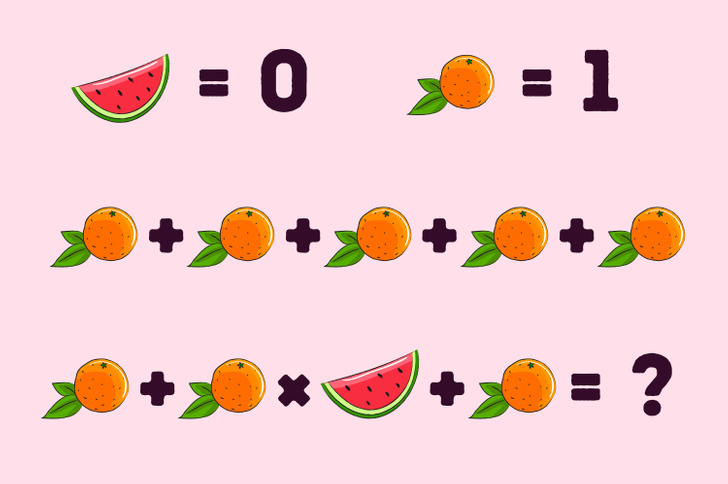
Look at the picture above and try to solve the equation as fast as possible. It’s very likely that you will make a mistake. Why?

If you replace oranges and the watermelon with numbers, you’ll get 1 + 1 × 0 + 1 = 2. Perhaps some of you got 7 as the result because you took the first line into your calculations. In fact, if all the oranges and watermelons were a part of one equation, the second line would start with a plus sign. That’s why we should only sum up and multiply the objects located in line 2.