How to Explain Percentages to a Child
Percentages can not only be complicated for children but also for adults. Because if you follow the school tips but don’t have a pen and paper at hand, you may experience difficulties when you need to make a quick calculation.
5-Minute Crafts would like to tell you how to easily and quickly explain percentages to your child, and how to do calculations in your head with the help of certain tricks, which school teachers will never tell you.
What a percentage is
Let’s say we have a huge square cake. It can be divided into 100 identical pieces. We can show it as a large 10 × 10 square.
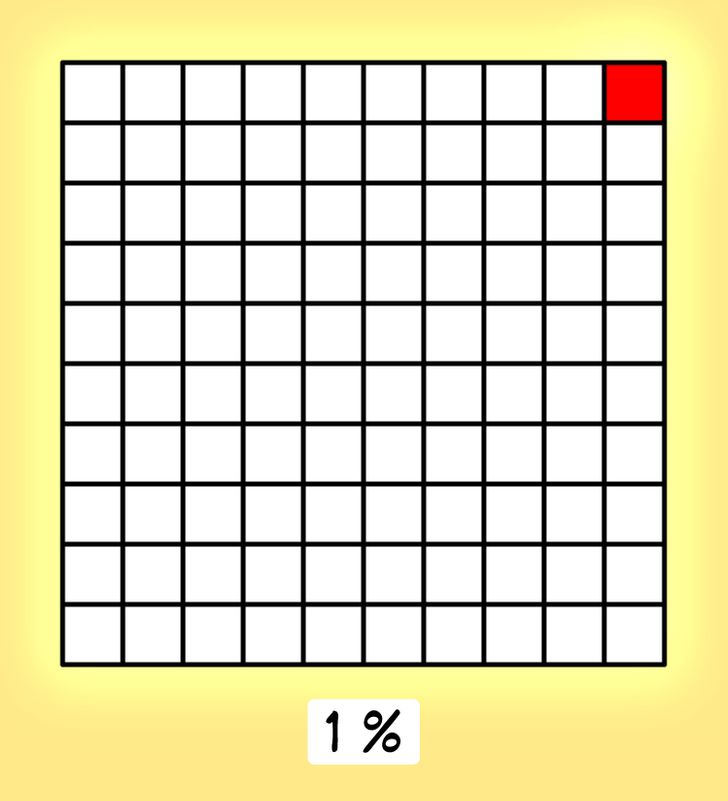
Let’s color in 1 cell, which symbolizes 1 piece. This is 1 percent (1%) of the whole cake, since the whole cake is 100%.
Thus, speaking of percentages, we think of a number as a whole, divided into 100 equal parts. One hundredth of this whole is 1%. In other words, dividing any number by 100, we get 1% of that number (for example: If we divide 5 by 100, we’ll get 0.05 which is 1% of 5).
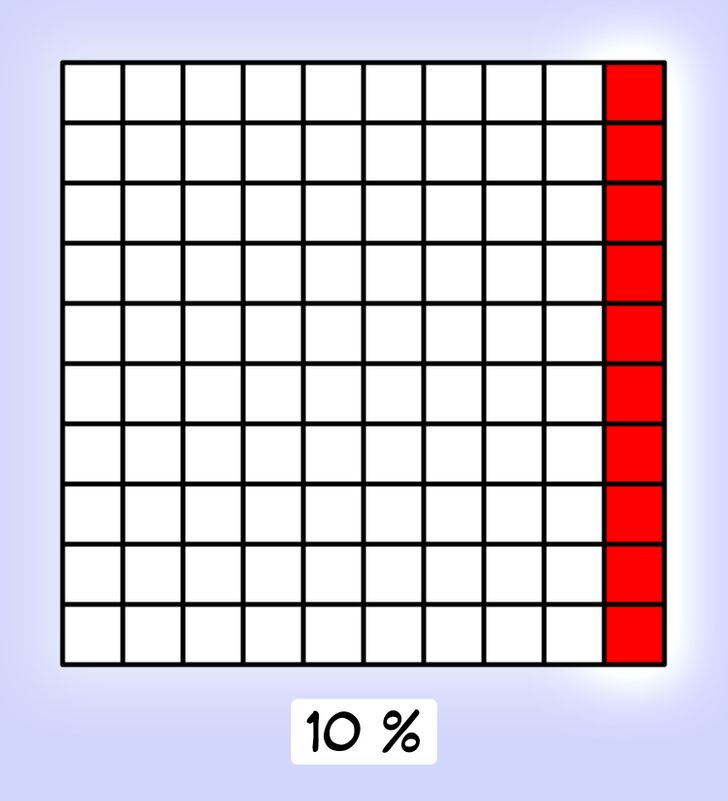
With the help of a 100-cell square, it’s convenient to learn the concept of percentages. Color in 10 cells: these are 10 pieces, or 10% of the whole cake.
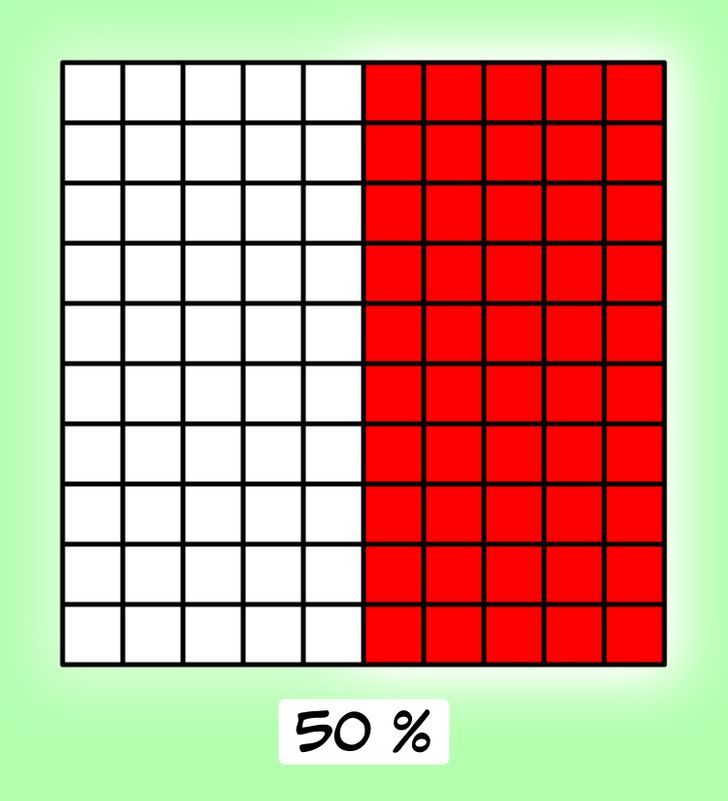
Now let’s color in half of the whole square, or 50 cells. These are 50 pieces, or 50% of the whole cake. Similarly, you can represent any percentage of an integer.
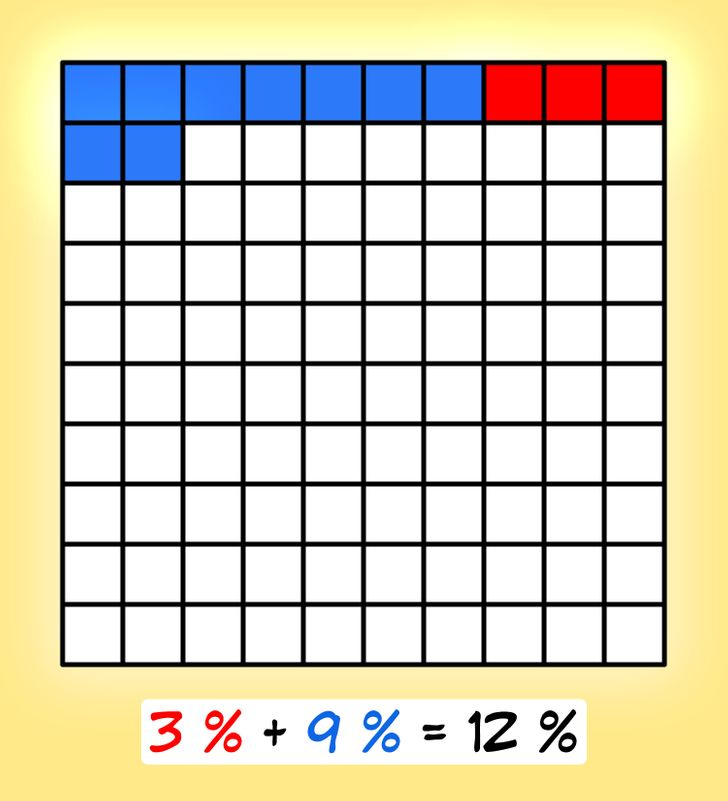
✅ Tip: Use this 10×10 square to display numbers as percentages and compare them with each other. It will also make it convenient to perform simple addition and subtraction with percentages.
Example: In the picture above, there are 3 red cells, corresponding to 3%, and 9 blue cells, corresponding to 9%. By counting all the painted cells or adding the numbers, we get 12%.
How to calculate percentages
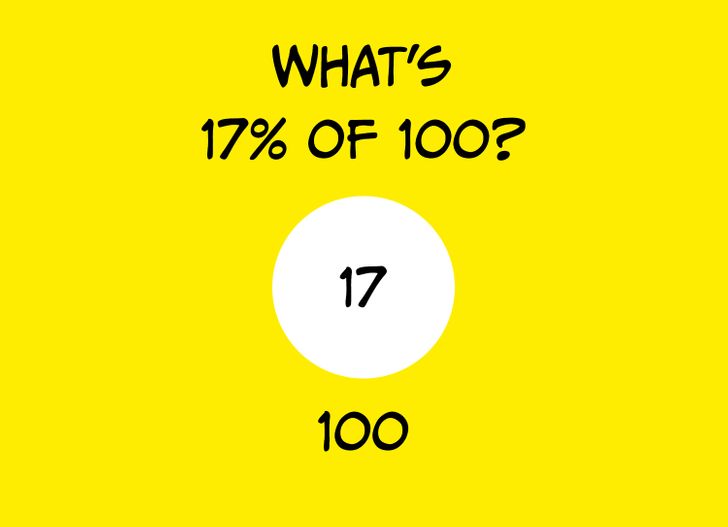
The picture above shows an elementary task. It’s easy to solve: if 1% is one hundredth of the number, then 17% of 100 is 17.
But what if you need to find a percentage of a different number? For example, 17% of 400.

You can use a simple trick and represent the number 400 as 4 hundreds. So we consider 17% of 400 as 17% of each hundred included in 400. Now you can just add them together and get the correct answer:
17 + 17 + 17 + 17 = 68
Now we know that 17% of 400 is 68. But this trick isn’t always helpful.
Example: You need to calculate 14% of 550.
From the definition of percentage, we know that 1% is one hundredth of a whole number (divided into 100 equal parts). So, in order to find the percentage of a number, you need to divide this number by 100 and multiply the result of the division by the number of percent. In this case, we need to do 2 actions:

The result is that 14% of 550 is 77.
In some tasks, the situation can be reversed, like when it’s necessary to solve for 100%.
Example: How to find 100% when 65% is 260?
Here we will make calculations in the opposite direction: first we solve for 1%, and then we calculate 100%. So, we need to do the following:

The result is that 100% equals 400.
This way you can solve various tasks with percentages, and usually this is the way they teach us to calculate percentages at school. But there are some tricks and techniques that will make this process much easier.
Trick 1: Swap numbers and percentages
For any problem, the following rule is true: X% of Y = Y% of X. Then you can swap the numbers and percentages if this makes the solution of the problem simpler.
Let’s say you need to calculate 4% of 75:

Let’s swap numbers and percentages, and then solve the problem.
If 4% of 75 = 75% of 4,
then we count 75% of 4 and get 3.
So 4% of 75 is also 3.
Thanks to this trick, in some cases, percentages can be calculated in your head.
Example: You need to calculate 18% of 50. Swap the numbers and calculate 50% of 18. The result is 9. So, 18% of 50 is also 9.
Trick 2: Calculate percentages as segments of a circle
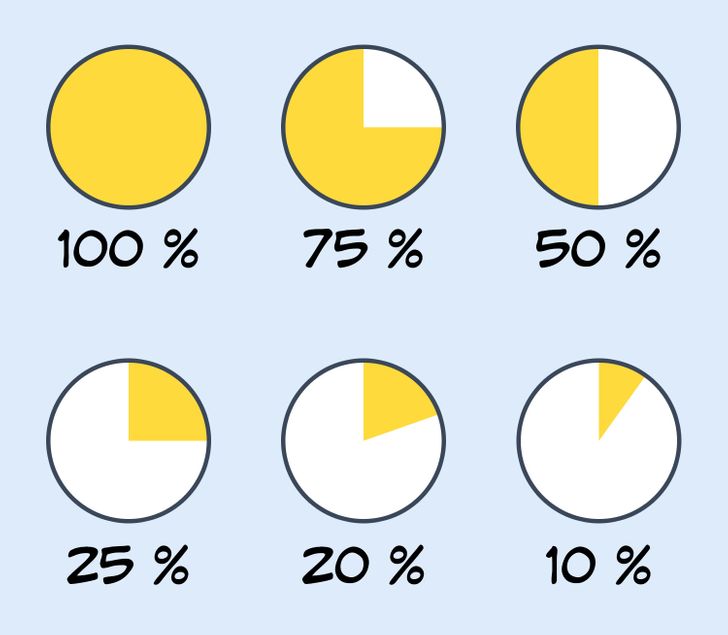
Look at the pie charts above: they show percentages as segments of a circle. For example, 50% is half a circle, 25% is a quarter, and 20% is one fifth.
So, in order to calculate 50% of the number, it’s enough to divide it by 2, and in order to calculate 20% of the number, you need to divide it by 5. Then, following this logic, you can make the following table:
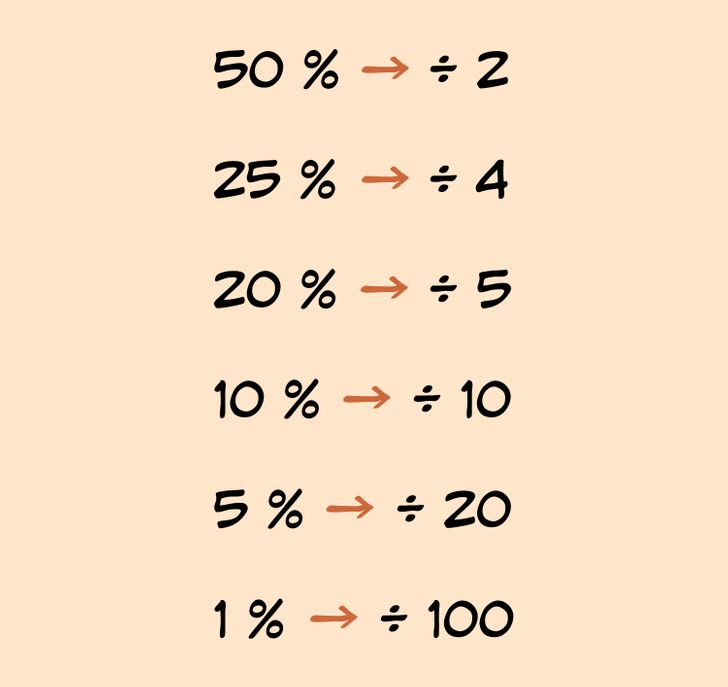
On the right, you can see the numbers by which the number must be divided when calculating a specific percentage of that number. Thus, you don’t have to look for a value, for example 20%, and then multiply it by something, where there’s a risk that you may confuse numbers and percentages. One action is enough.
More complex problems can be solved in a similar way. For example, 16% of 42 can be represented as 10% + 5% + 1%, that is 4.2 + 2.1 + 0.42 = 6.72.
Trick 3: Move a decimal point to calculate percentages
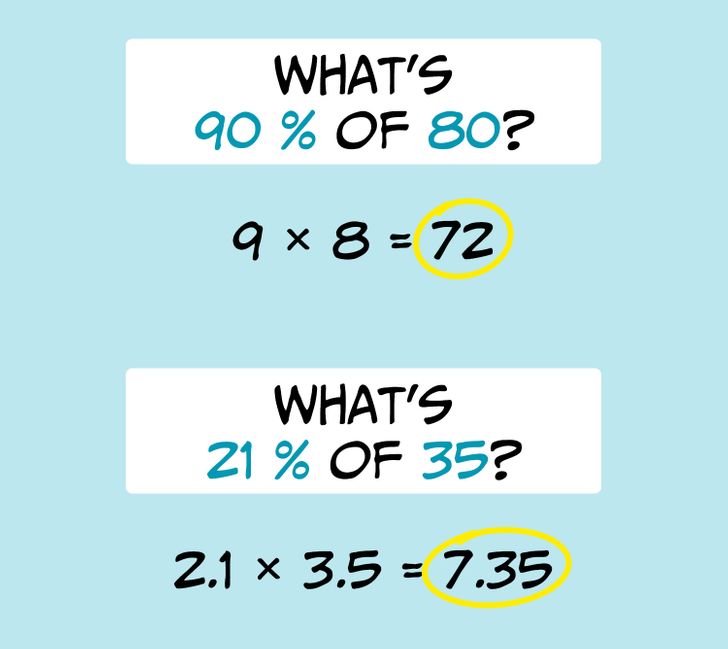
It’s simple: move the decimal point by 1 digit to the left in each number, and then multiply these numbers. For example, to calculate 90% of 80, multiply 9 by 8 and get 72. Similarly, if you need to find 21% of 35, multiply 2.1 by 3.5 and get 7.35.
Real life percentages calculation examples
If at school it is required to solve examples in a notebook, in everyday life, tasks for children can be quite simple, but they will allow them to calculate percentages in their head in the simplest possible ways.
For example:
- To calculate 10% of 4 kg, it is enough to remember that 10% is one tenth of a hundred, so you can divide 4 kg by 10. You get 0.4 kg, or 400 g.
- To calculate 5% of 5 meters, you can imagine 5 m as 500 cm. Then divide 500 by 10%, getting 50. This result should be halved to get 5%. Thus, 5% of 5 m is 25 cm, or 0.25 m.
- To calculate 40% of $15, it’s enough to calculate 10% of $15 first, which is $1.5, and then multiply it by 4. So, 40% of $15 is $6.
Having mastered this approach, a child will be much better adapted to situations where the ability to calculate percentages will help them choose a more advantageous offer when they are adults.

Task 1. 2 identical headphones are sold in 2 online stores. At store 1, they cost $45, but the seller promises free shipping worldwide. At store 2, the price is the same, but they give you a 15% discount, however, you will have to pay $8 for delivery. At which store is it more profitable to buy headphones?
15% is 10% + 5%. It’s easy to calculate that 10% of $45 is $4.5. Thus, 5% is $2.25. Add 4.5 and 2.25 to get 6.75. So, 15% of $45 is $6.75. This means that you will save $6.75 at store 2, but you will still spend more on the delivery, which is $8. So, it’s more profitable to buy the item at store 1.

Task 2. You find an excellent TV set in a store window with a price tag of $2,600. Today there is a sale that gives a 25% discount on all items. You have only $2,000. Do you have enough money to buy the TV set?
We know that 25% is one quarter of 100%. Thus, you need to find out how many dollars are 75%, or 3 quarters. To do this, you have to divide the price without the discount into 4 parts, and then multiply by 3:
2600 ÷ 4 = 650
650 × 3 = 1,950
So, the price of the TV set, taking into account the discount, is $1,950, which means that you can afford it.
Task 3. You’ve come to a store to buy sneakers. Their price is $376. You get a special offer for an 11% discount, but if you use a store card, the sneakers will cost $320. Which option is more profitable?
Let’s see how many dollars 11% are. Let’s take the price of the sneakers and calculate 1% by moving the decimal point in 376 2 digits to the left. Thus, 1% is $3.76, and 10% is $37.6 respectively. Let’s add them up and get $41.36.
We could calculate this in a different way, by using trick 3 and simply moving the decimal point in the both numbers, and then multiplying them:
1.1 × 37.6 = 41.36
Now we subtract this number from the usual price of the sneakers. Taking into account the special offer, the sneakers will cost $334.64, which means that the purchase will be profitable if you use the store card and buy them for $320.