Parts of a Circle, and How Different They Are
We often come across circles in our everyday lives, even though we may not always notice them. For example, if you know the diameter of a tire, you can find out the distance it covers within one turn. Or if you know the radius of a pool, you can calculate the area it will cover in your garden.
5-Minute Crafts wants to help you figure out what a circle is and what the differences between its parts are.
What a circumference is

If you take many points and place them one after another, you’ll get a line. If it has no curve in it, has no beginning or end, and continues indefinitely in both directions, then this is a straight line. If the line bends due to a specific location of the points, then it’s a curve. The ends of the curved line may not connect, forming an open curve. When they are connected, you’ll get a closed curve.
Draw a closed curved line so that all its points are in the same plane and at an equal distance from a given point, which is located in the same plane, and you’ll get a separate geometric shape called a circle. If you take the plane inside this circle, you’ll get another geometric shape called a disc.
You need a compass to draw a circle. It’s a special instrument that allows you to inscribe circles and arcs.
What parts of a circle there are
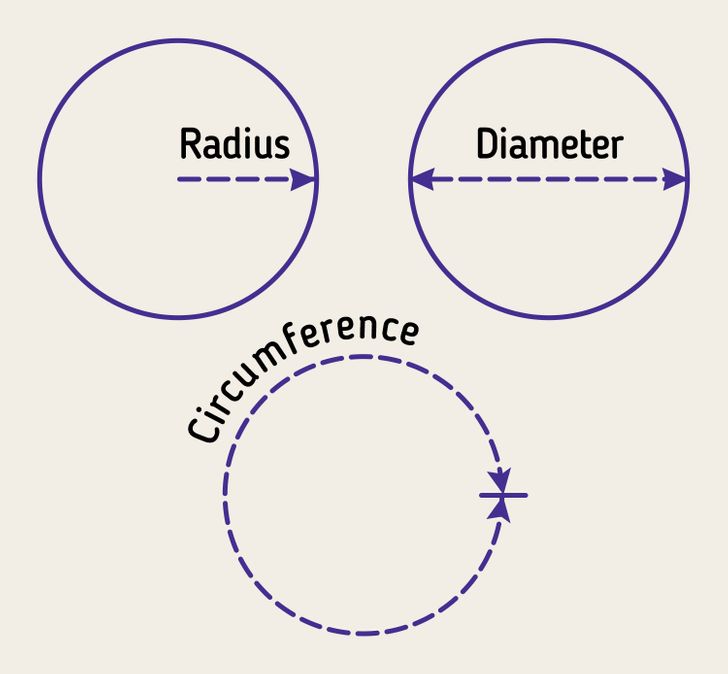
- The length of a line segment from the center of the circle to its perimeter is always the same and is called a radius.
- A line segment that connects any 2 points on the circle and passes through the center of the circle is called a diameter. Also, the diameter of a circle is always twice its radius.
- If you measure the length of a closed curve that binds a circle, you’ll get the circumference. You may also say that the circumference of a circle is a special case of the perimeter if we consider the circumference as the boundary of a circle.
Let’s use the letter “R” for radius, the letter “D” for diameter, and the letter “L” for circumference. As mentioned above, the diameter of a circle is always twice its radius:
D = 2R
If you divide the circumference by the diameter of the circle, you’ll always get the same number, pi (π). It is a mathematical constant, an irrational number, and it has an infinite number of digits after the decimal point:
π ≈ 3.1415926535897932384626433832795...
Thus, to find the circumference of a circle, you need to multiply the number π by the diameter or by 2 radii of the circle:
L = π × D = π × 2R
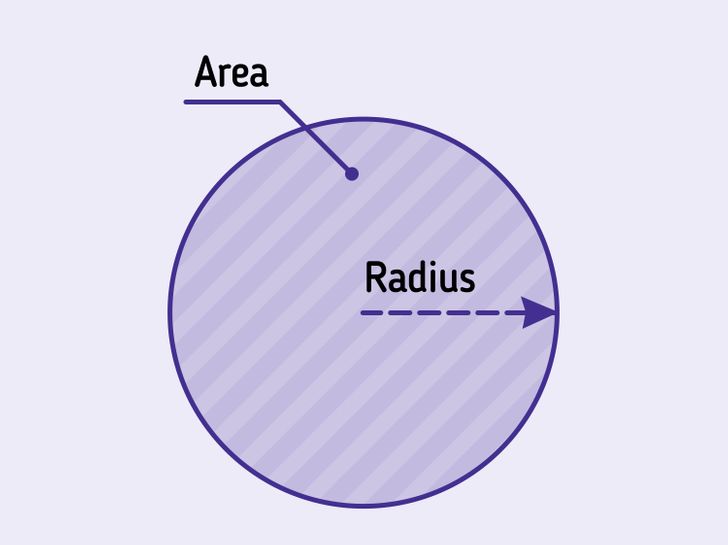
If you know the length of a radius, it’s easy to calculate the area of a circle. You just need to multiply the number π by the square of the radius of a circle:
S = π × R2 = (π × D2) : 4

- A line segment connecting any 2 points on a circle is called a chord.
- A straight line that connects any 2 points on a circle is called a secant. Thus, a chord is always on a secant.
- If a straight line has only 1 common point with a circle, this line is called a tangent. It’s good to know that a tangent is always perpendicular to the radius drawn through the point of tangency. And vice versa: if a straight line passes through the radius endpoint located on a circle and is perpendicular to the radius, it’s called a tangent.
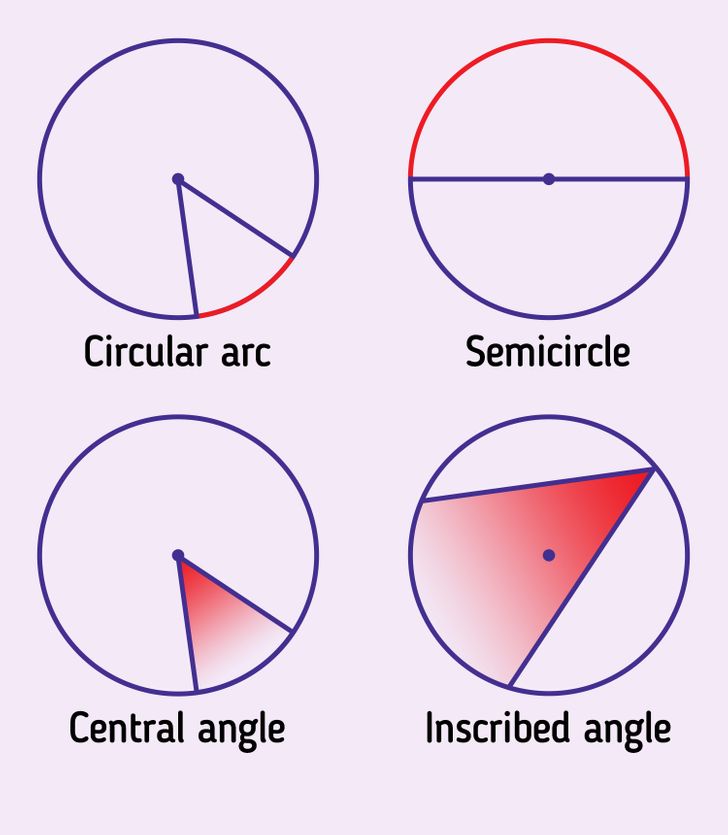
- The arc of a circle is called a circular arc. It’s located between any 2 points on a circle.
- An arc can be a semicircle if a line segment that connects its endpoints is the diameter of a circle.
- If you draw line segments from each endpoint of an arc to the center of a circle, you’ll get an angle with a vertex at the center of a circle, which is called a central angle.
- If an angle is formed in the interior of a circle when 2 chords intersect on the circle, such an angle is called an inscribed angle.
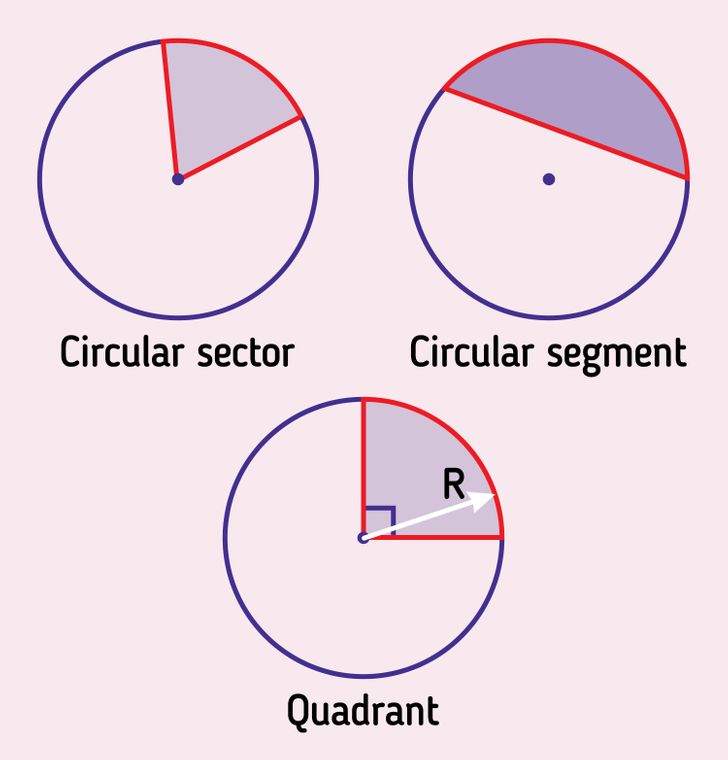
- The part of a circle that lies within a certain central angle is called a circular sector.
- The part of a circle that is between an arc of a circle and its chord is called a circular segment. If you draw a perpendicular from the middle of the chord to the arc itself, you’ll get the sagitta, which is also called the height of an arc.
- If 2 radii form an angle of 90°, then the circular sector they bind is called a quadrant.